Why this roll-down test quickly went downhill
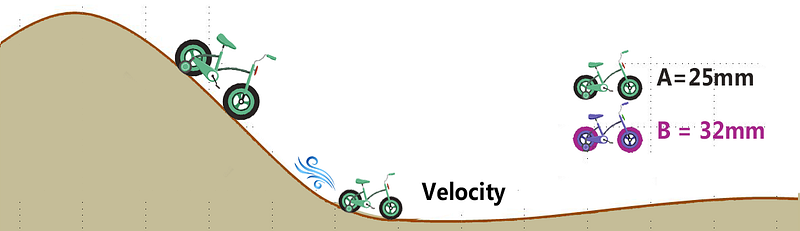
David Arthur of cycling weekly recently did a roll down on youtube. It was a pretty nice attempt at face value and it makes interesting viewing. He did six runs 3 with a 25mm and 3 with a 32mm on a 5% downhill over around 500m (25m vertical drop) and he found the 32mm was quite a bit faster at the bottom of the hill. Before I say more, credit to David Arthur for trying to find an answer to this qq.
Watch it and see what you think…..what does it show? Actually I’ll save you time he found the 32mm was “faster”…..
the 32mm was 0.7kph or 3% faster than the 25mm
Case closed? Haha, not quite. In fact, case open.
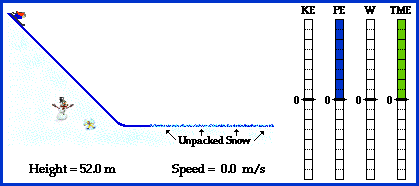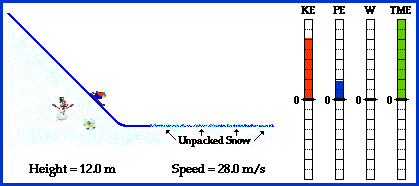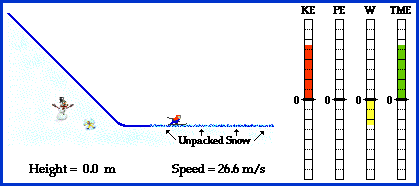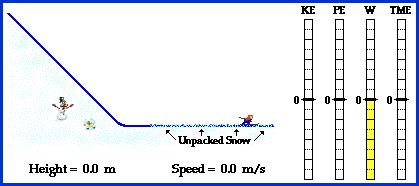
Conservation of Energy: Roll Down and Roll Up Test!
Pretend the bike was hanging on a rope and swung down the hill, like a pendulum. Provided it didn’t hit anything it would swing up the other side just as far right?
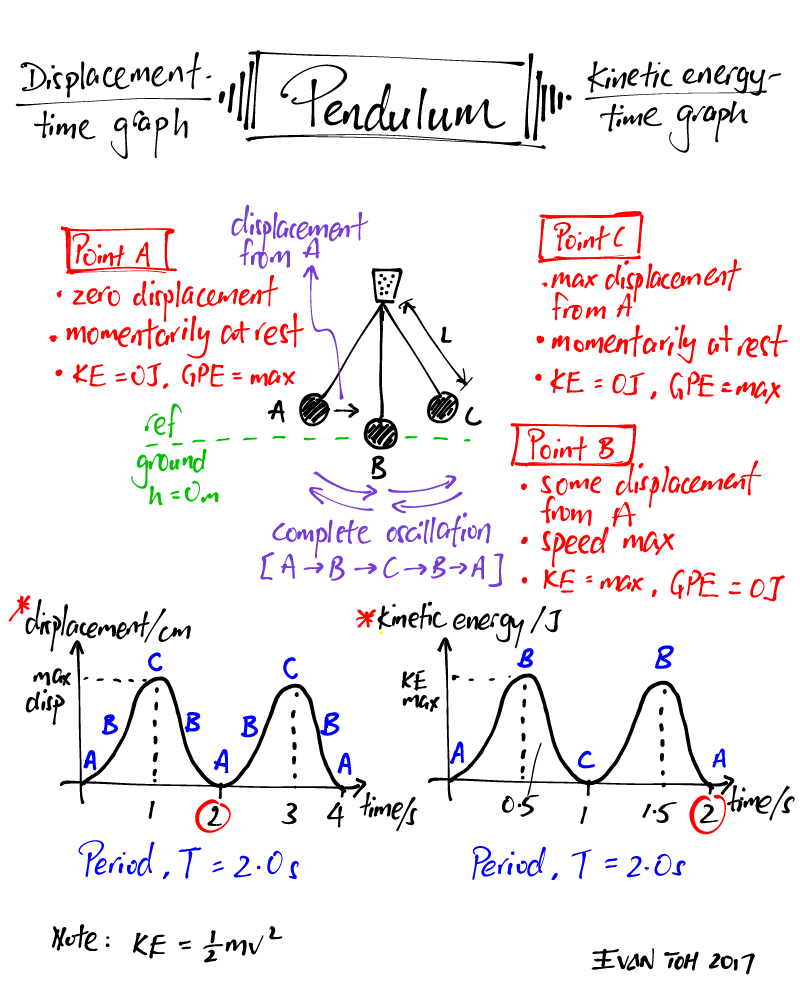
Pretty much, you don’t need fancy maths to know:
the law of the conservation of energy tells us that energy is neither created nor destroyed. It can only transition from one form of energy to another. Mechanical energy (ME) is the total energy of our pendulum and is the addition of gravitational potential energy and kinetic energy
And in a roll-down test, it’s pretty much the same, if *nothing got in the way* in a roll down test, it would be pretty sweet to have the bike roll down one side and up the other side because it would go up *almost* the same distance.
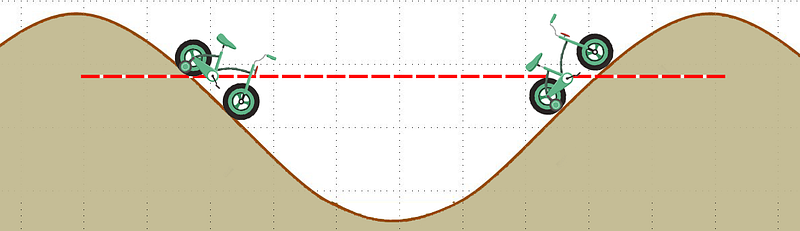
Except it wouldn’t *quite* go up the same distance because something would have robbed the bike of energy…..namely:
Air resistance & wind (slowing the bike)
Rolling resistance
(and a tiny bit of friction)
Now let’s say you rolled down Bike A with 32mm tires and Bike B with 25mm
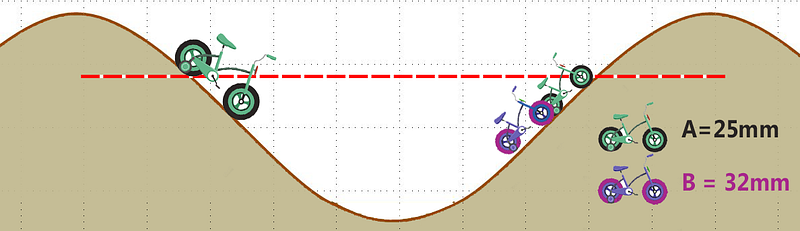
From the same starting position, in the same conditions, one might consistently roll up a little lower on the other side, and this one would be the one losing more to CRR or aero.
Because the differences would be tight, its best to do the test maybe 6,8 or 10 times, alternating the order (to minimize the effect of outside conditions):
25mm /32mm/25mm /32mm/25mm /32mm/25mm /32mm
If you didn’t alternate then there is a high chance changes in wind (or maybe temp) would destroy the results completely.
1/2 a test = Doh!
Unfortunately, this is not what people doing roll-down tests do. What they do is something like this, they chose a downhill only, and measure either speed at the bottom, or distance before the bike stops.
They also forget to randomize the order, and usually don’t do enough runs. They probably forget to control tire pressure which would be really interesting if they did this:
25mm 80psi /32mm 80psi /25mm 80psi /32mm 80psi/
25mm 60psi /32mm 60psi /25mm 60psi /32mm 60psi/
If you want, you can stop reading there, this type of roll down test is *very unlikely* to conclude anything…….
If you want to go into nerd mode, we can look at why velocity/distance roll-down tests don’t work very well….and to do this we need to go back to school! sorry!
Physics of Falling and Rolling Down an Incline
An counterintuitive fact is that when free falling, sliding or rolling downhill mass of the object makes no difference to acceleration in a vacuum.
a 1000-kg baby elephant and a 1-kg overgrown mouse…fall at the same rate…
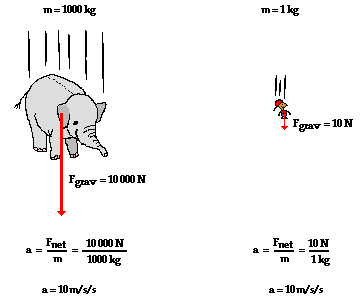
So mass doesn’t matter? Not quite, mass does affect the kinetic energy and the moment of inertia but not overall acceleration.
However, with a rolling object it more complicated because a rolling object will take time to get going if its mass it near the edges. A solid disc will roll down faster than a cylinder shape (aka torroid aka wheel/tire) share.
Here is an animation……and an explanation (LINK)
The initial kinetic energy is zero. The final kinetic energy consists of translational and rotational parts: Mgh = ½ Mv2 + ½ Iω2
The more the mass of the object is at its edges, the slower it will roll. For a 25mm tire 93% of the mass is at the edge (its 93% open space) and for a 32mm its 91% so this suggests a 32mm will roll accelerate slightly faster.
Kinetic Energy and Drag
Wait….you probably spotted we don’t ride in a vacuum. If you drop a bowling ball and a basket ball from a tall building they initially accelerate with the same speed but at the bottom there is a difference….and this is due to the effect of air resistance (drag). Here is a clip from a video where they are both dropped at the same time at the top, at the bottom they land about 1m difference. Why? because of kinetic energy vs drag.

Remember that the equation for drag force (FD) is given by
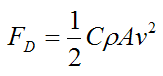
C is the drag coefficient
ρ is the density of the air
A is the projected frontal area perpendicular to the flow direction (that is, perpendicular to velocity v)
v is the velocity of the relative to the air
Now drop a ping-pong ball and it will reach terminal velocity within about 4m due to its punny kinetic energy relative to massive air drag….
The bowling ball has large kinetic energy. At the top of the hill (or building), all the energy is in the form of potential energy (mgh m=mass, g=acceleration of gravity, and h=height). At the bottom of a hill, the energy is all kinetic, represented as KE = 1/2 mv².
mgh = 1/2 mv² + air drag
mgh = 1/2 mv² + 1/2 CPAv²
mgh = 1/2 v² (m+CPA)
v² = 2mhg /(m+CPA)
v² = 2mhg /(m+CPA)
v=sqrt (2hg)….without drag
v=sqrt (2mgh/(m+CPA))….with drag
Look at this another way, a train weighing 1000 tonnes and a bicycle weighing 80kg are both travelling at 30kph down 5% slope (without any power) which will travel furthest on the flat? The train of course, all that massive kinetic energy has to be overcome by drag. Here is a nice illustration in a skier…
So it turns out the elephant and the mouse initially fall together but the mouse quickly reaches terminal velocity within about 20m and survives the fall, whereas the elephant might accelerate for another 100 vertical metres.

…….Back to the Tire and the Roll-Down Test from the video

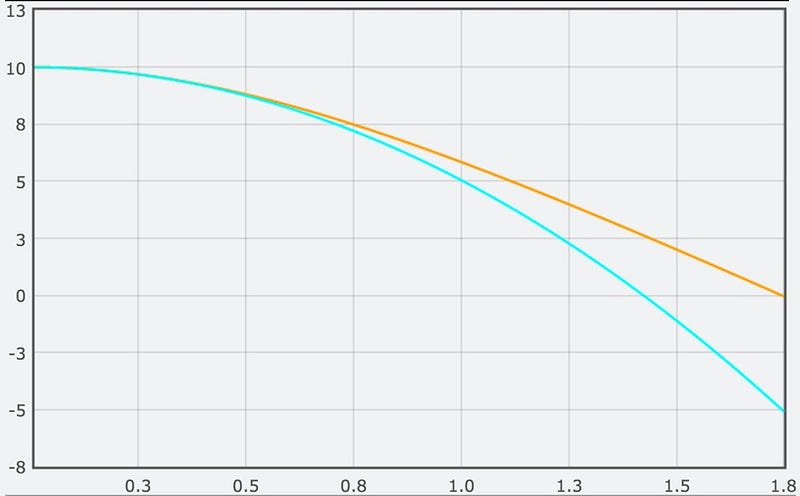
So back to our two tires…25mm vs 32mm, look at this chart….
CONCLUSION: DIFFERENCES DUE TO PHYSICS NOT CRR
A. 32mm wheels/tires accelerate slightly faster than 25mm tires due to difference in torroid shape (91% vs 93%)
B. 32mm wheels/tires have higher kinetic energy than 25mm (even at the same speed) although this difference is about 0.1% of the whole
C. 32mm tires have slightly higher aero drag than 25mm tires…how much? we worked it out here: 32mm area 0.055 vs 25mm area 0.046…..effect 1watt at 24kph (fft.tips/tyre).
You can plug this all into OMNI calculator (here…reset to torroid) and they suggest that 32mm will roll down a 25 metre incline 2.1kph faster than a 25mm tire (but they don’t control for air resistance difference, which I would say cut that in half).
The above video found a 32mm was 0.7kph or 3% faster at the bottom of the hill which is in line with the above calculations and nothing to do with CRR.
SUMMARY
What David Arthur of cycling weekly has shown, is that physics still applies and very little about the tire properties. Any gains demonstrated with the 32mm would be lost when going uphill.
What he was trying to find out was whether rolling resistance properties made a difference. A better experiment would be to measure how fall up the other side of the hill the bike climbed (without power)…ie roll down and roll up. This would effectively control for tire weight and torroidal properties and any differences would be due to CRR and aero drag which is what we wanted to know.
So sorry David your nice experiment is flawed. But still a nice effort and an interesting discussion!
